零知识证明 - PLONK算法零知识性问题

前几天，看到PLONK算法作者Ariel Gabizon发了一个Twitter，提到原始PLONK算法的零知识性存在问题：

简单的说，原始论文把商多项式t，直接分成三部分t_lo，t_mid，t_hi，并没有在这三部分多项式添加随机性。这样的话，Simulator（模拟器）不能模拟出这些多项式的承诺。

理解这个问题，先理解一下模拟器：

Formally, what it means for a protocol to be zero-knowledge is defined in terms of a simulator. The simulator is a machine that operates in a different world that is indistinguishable from the real-world for the party that is assessing whether knowledge was leaked. In this world, called the ideal world, the simulator has additional powers that allows it to compute a proof without knowing anything besides the truth of the statement.

模拟器（simulator）用来证明零知识性的。Simulator和现实世界不一样，拥有除了witness外的其他所有知识。如果Simuator能构建能被验证者验证的证明，说明一个证明能在没有witness信息的情况下构建。反过来说，如果Simulator能“模拟”出一个正确的证明，则该证明系统是零知识的。

体会Simuator，可以再看看Groth16算法的Simulator：

在不提供witness的情况下，随机出A/B，可以通过公开输入信息以及trusted setup的信息计算出C，从而构建正确的证明。

理解了Simulator，再看PLONK原始论文的零知识性。因为t_lo，t_mid，t_hi并没有添加随机性，Simulator为了构造这些多项式的Commitment，必须通过商t多项式计算。要构造商t多项式，需要witness信息。所以说，PLONK原始论文中的算法不能构造相应的Simulator。进一步说，因为原始PLONK算法不具备零知识性，witness有可能泄漏。

Ariel Gabizon也在Twitter中给出了更新后（添加了随机性）的t_lo，t_mid，t_hi的计算方法，并强调调整后算法的Simulator也会补上。

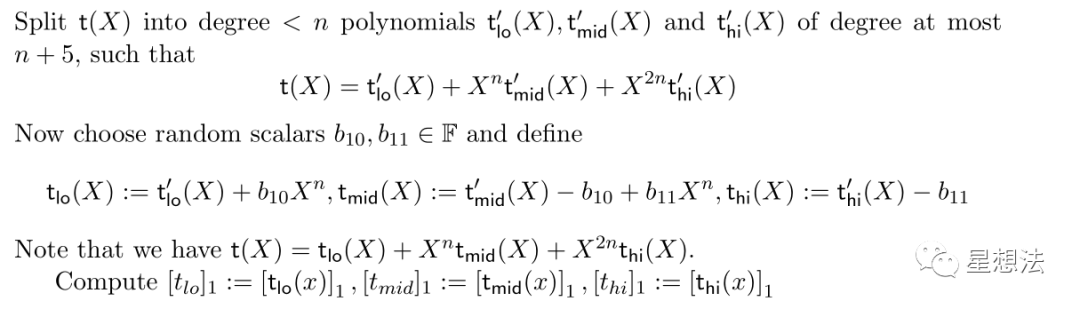
总结：

PLONK的原始论文存在一个零知识性的问题。商t多项式没有添加随机性，导致simulator不能模拟出证明。

本文首发于：https://mp.weixin.qq.com/s/AaczgL2wqiLp1zUITlGEjQ