1. 海绵结构
2. 轮函数（常量加、非线性变换、线性变换） 2.1 Full Rounds变换 2.2 Partial Rounds变换 2.3 Permutation全流程
3. Poseidon的吸入、置换、压缩
4. Goldilocks域 & Plonky2中Poseidon的优化 4.1 Poseidon的MDS运行过程 4.2 Poseidon在Goldilocks域上的运行过程

1. 海绵结构

Poseidon哈希函数采用下面的海绵结构(Sponge Construction）来进行设计，其中P是Posedion每轮的置换函数，$I$是初始状态。假设初始状为$I$为全$0$向量，有一个消息$m=m_1||m_2||m_3||m_4$，输出为$h=h_1||h_2$，且每个$m_i$和$h_i$都是$r$位长度的，那么他们将经过以下模式来进行计算：

1. $I$首先被赋值为$m_1$，并经过置换$P$，得到第一轮的长度为$r$的输出结果，随后和$m_2$进行异或，得到下一轮结果；
2. 循环往复，直到输入$m_4$，随后输出前$r$位结果$h_1$，并将$h_1$再经过一次置换，得到结果$h_2$；
3. 将$h=h_1||h_2$作为Poseidon hash的输出。

2. 轮函数（常量加、非线性变换、线性变换）

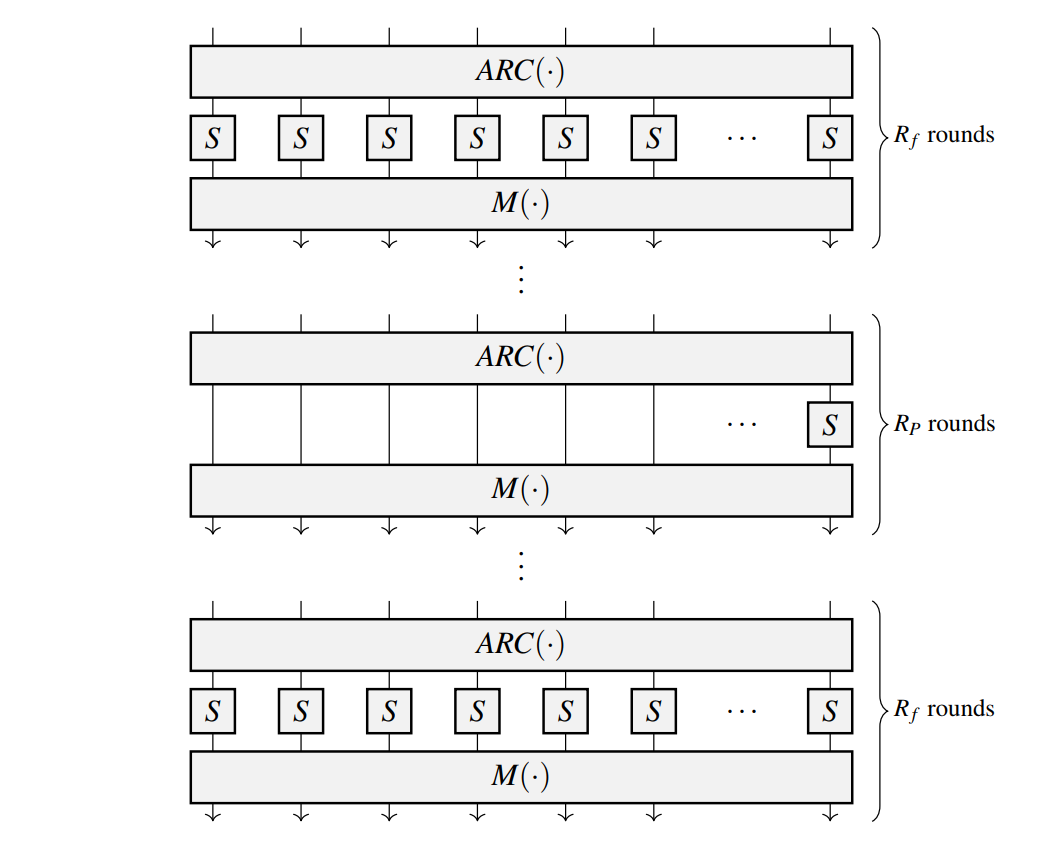
下图是Poseidon哈希函数对应的置换函数的基本流程，其中包含$R_f$轮的full state和$R_p$轮的partial state，其中$R_f$轮需要所有状态均经过$S$盒(S-Box)的置换，$R_p$轮只需要经过一个$S$盒的置换即可，图中的术语如下所示

1. AddRoundConstants, denoted by ARC(·)， 线性层，对每个codeword加上一个预先定义的数字；
2. SubWords, denoted by S-box(·) or by SB(·)， 非线性层，对每个codeword进行幂次运算，一般是$X^3$或$X^5$。其中，$X$是每个codeword。Plonky2采用$X^7$；
3. MixLayer, denoted by M(·)， 线性层，和预先定义的矩阵进行乘法操作。

2.1 Full Rounds变换

rust
    #[inline]
    fn full_rounds(state: &mut [Self; SPONGE_WIDTH], round_ctr: &mut usize) {
        for _ in 0..HALF_N_FULL_ROUNDS { // 经过constant layer, SBOX和MDS
            Self::constant_layer(state, *round_ctr);  // 加上一个数字, 线性层
            Self::sbox_layer(state); // X^3或X^5, 非线性层
            *state = Self::mds_layer(state); // 矩阵乘法, 线性层
            *round_ctr += 1;
        }
    }
    // plonky2/src/hash/poseidon.rs

上述代码展示了Poseidon hash的full rounds层对应的变换

2.2 Partial Rounds变换

rust
    fn partial_rounds(state: &mut [Self; SPONGE_WIDTH], round_ctr: &mut usize) {
        Self::partial_first_constant_layer(state);
        *state = Self::mds_partial_layer_init(state);

        for i in 0..N_PARTIAL_ROUNDS {
            state[0] = Self::sbox_monomial(state[0]);
            unsafe {
                state[0] = state[0].add_canonical_u64(Self::FAST_PARTIAL_ROUND_CONSTANTS[i]);
            }
            *state = Self::mds_partial_layer_fast(state, i);
        }
        *round_ctr += N_PARTIAL_ROUNDS;
    }
        // plonky2/src/hash/poseidon.rs

上述代码展示了Poseidon hash的partial rounds层对应的变换

2.3 Permutation全流程

rust
    #[inline] // Poseidon hash的全流程
    fn poseidon(input: [Self; SPONGE_WIDTH]) -> [Self; SPONGE_WIDTH] {
        let mut state = input;
        let mut round_ctr = 0;

        Self::full_rounds(&mut state, &mut round_ctr);
        Self::partial_rounds(&mut state, &mut round_ctr);
        Self::full_rounds(&mut state, &mut round_ctr);
        debug_assert_eq!(round_ctr, N_ROUNDS);

        state
    }
    // plonky2/src/hash/poseidon.rs

上述代码展示了Poseidon hash中对应的一轮permutation的全过程

3. Poseidon的吸入、置换、压缩

完整的Poseidon Hash结构如下所示：

• 吸收阶段（Absorb Phase）: hash_n_to_hash_no_pad 函数负责读取输入数据，并通过与内部状态 XOR 后执行置换。

rust
    fn hash_no_pad(input: &[F]) -> Self::Hash {
        hash_n_to_hash_no_pad::<F, Self::Permutation>(input)
    }
    // plonky2/src/hash/poseidon.rs

• 置换阶段（Permutation Phase）: PoseidonPermutation 对应 P，实现了每一轮置换，涵盖了全轮（Full Rounds）和部分轮（Partial Rounds）的步骤。

rust
pub struct PoseidonPermutation<T> {
    state: [T; SPONGE_WIDTH],
}
// plonky2/src/hash/poseidon.rs

• 压缩阶段（Squeeze Phase）: 压缩阶段从内部状态中提取哈希结果。

rust
/// A one-way compression function which takes two ~256 bit inputs and returns a ~256 bit output.
pub fn compress<F: Field, P: PlonkyPermutation<F>>(x: HashOut<F>, y: HashOut<F>) -> HashOut<F> {
    // TODO: With some refactoring, this function could be implemented as
    // hash_n_to_m_no_pad(chain(x.elements, y.elements), NUM_HASH_OUT_ELTS).

    debug_assert_eq!(x.elements.len(), NUM_HASH_OUT_ELTS);
    debug_assert_eq!(y.elements.len(), NUM_HASH_OUT_ELTS);
    debug_assert!(P::RATE >= NUM_HASH_OUT_ELTS);

    let mut perm = P::new(core::iter::repeat(F::ZERO));
    perm.set_from_slice(&x.elements, 0);
    perm.set_from_slice(&y.elements, NUM_HASH_OUT_ELTS);

    perm.permute();

    HashOut {
        elements: perm.squeeze()[..NUM_HASH_OUT_ELTS].try_into().unwrap(),
    }
}
// plonky2/src/hash/hashing.rs

rust
pub fn hash_n_to_hash_no_pad<F: RichField, P: PlonkyPermutation<F>>(inputs: &[F]) -> HashOut<F> {
    HashOut::from_vec(hash_n_to_m_no_pad::<F, P>(inputs, NUM_HASH_OUT_ELTS))
}
// plonky2/src/hash/hashing.rs

compress和hash_n_to_hash_no_pad实现了具体的吸收（absorb）和压缩（squeeze）。hash_n_to_hash_no_pad 会调用 PoseidonPermutation 进行内部状态的置换，并在合适的时间点进行数据的压缩。

特例：two_to_one 函数实现了类似 Merkle 树节点的哈希，它将两个哈希值（即两个叶子节点）进行合并，并生成新的哈希值，主要用于FRI构造Merkle Hash调用Poseidon Hash的过程。

rust
    fn two_to_one(left: Self::Hash, right: Self::Hash) -> Self::Hash {
        compress::<F, Self::Permutation>(left, right)
    }
    // plonky2/src/hash/poseidon.rs

4. Goldilocks域 & Plonky2中Poseidon的优化

在Plonky2中，一般选取Goldilocks域作为有限域$F_p$，其中$p=2^{64}-2^{32}+1$，它可以将任何的witness/codeword转换为$64$位长的数，其主要具有以下优点：

1. 目前的处理器处理$64$位长的数十分高效，因为它正好可以使用传统的bit string的处理方式来处理算术运算，比如乘以$2$或者除以$2$相当于移位操作；
2. 在编写为算术电路时，因为Goldilocks域的操作均属于算术操作，所以无需对其进行模拟，相比于传统的基于二进制的SHA256哈希函数而言，该类哈希函数具有更小规模的电路和更少的约束个数。

Plonky2在MDS层使用的矩阵的第一行为$[1; 1; 2; 1; 8; 32; 2; 256; 4096; 8; 65536; 1024]$，其中每个元素都是2的幂次方，它使得算术电路中的乘法操作和二进制中的移位操作等价，极大地提高了运算效率，除此之外，矩阵中的每个元素值都比较小。

4.1 Poseidon的MDS运行过程

rust
fn mds_layer(state: &[Self; 12]) -> [Self; 12] {
        let mut result = [GoldilocksField::ZERO; 12];

        // Using the linearity of the operations we can split the state into a low||high decomposition
        // and operate on each with no overflow and then combine/reduce the result to a field element.
        let mut state_l = [0u64; 12];
        let mut state_h = [0u64; 12];

        for r in 0..12 {
            let s = state[r].0;
            state_h[r] = s >> 32;
            state_l[r] = (s as u32) as u64;
        }
                // 使用优化的FFT进行运算
        let state_h = poseidon12_mds::mds_multiply_freq(state_h);
        let state_l = poseidon12_mds::mds_multiply_freq(state_l);

        for r in 0..12 {
            let s = state_l[r] as u128 + ((state_h[r] as u128) << 32);
            // from_noncanonical_u96将输入的 96 位数据（
           // (表示为一个 64 位的低位部分和一个 32 位的高位部分）转换为 Goldilocks 域
           // 中的一个元素
            result[r] = GoldilocksField::from_noncanonical_u96((s as u64, (s >> 64) as u32));
        }

        // Add first element with the only non-zero diagonal matrix coefficient.
        let s = Self::MDS_MATRIX_DIAG[0] as u128 * (state[0].0 as u128);
        result[0] += GoldilocksField::from_noncanonical_u96((s as u64, (s >> 64) as u32));

        result
    }
    // plonky2/src/hash/poseidon_goldilocks.rs

4.2 Poseidon在Goldilocks域上的运行过程

1. 初始化结果数组

rust
let mut result = [GoldilocksField::ZERO; 12];

• 这里初始化了一个长度为$12$的 result 数组，用于存储最终的 MDS 变换结果。初始值为 GoldilocksField::ZERO。

2. 分离状态向量的高位和低位

rust
let mut state_l = [0u64; 12];
let mut state_h = [0u64; 12];

for r in 0..12 {
    let s = state[r].0;
    state_h[r] = s >> 32;
    state_l[r] = (s as u32) as u64;
}

• state_l 和 state_h 数组分别存储了状态向量中每个元素的低位和高位部分。
• 通过位操作，state_h 存储元素的高$32$位，state_l 存储低$32$位。

3. 用FFT加速MDS算法

rust
let state_h = poseidon12_mds::mds_multiply_freq(state_h);
let state_l = poseidon12_mds::mds_multiply_freq(state_l);

• 这里调用 poseidon12_mds::mds_multiply_freq 函数对 state_h 和 state_l 分别基于 FFT的 MDS 矩阵乘法，以加速矩阵乘法运算。

4. 合并高位和低位的结果

rust
for r in 0..12 {
    let s = state_l[r] as u128 + ((state_h[r] as u128) << 32);
    result[r] = GoldilocksField::from_noncanonical_u96((s as u64, (s >> 64) as u32));
}

• 这里将高位和低位部分重新组合为$96$位整数。state_h 部分左移$32$位后与 state_l 相加，得到完整的$96$位数值。
• 然后调用 GoldilocksField::from_noncanonical_u96 将这个$96$位整数转换为 Goldilocks 域内的元素，并存储到 result 中。

5. 添加对角矩阵的一个非零元

rust
let s = Self::MDS_MATRIX_DIAG[0] as u128 * (state[0].0 as u128);
result[0] += GoldilocksField::from_noncanonical_u96((s as u64, (s >> 64) as u32));

• 最后，为了完成 MDS 变换的对角部分，函数将 MDS_MATRIX_DIAG[0] 和 state[0] 相乘后添加到 result[0] 中。